Math Coaching at EMES: The Sequel

Ellen Shedd's freshly renovated room at EMES is to die for.

A cancelation left me with an open week, so we carved out some time to work with the fifth grade teachers at EMES since our last attempt at a coaching week was foiled by snowfall and programming conflicts.  I had a blast (love those fifth graders!).

A couple of things I really love about EMES: the "Acts of Kindness" announcements and the gorgeous classrooms (see left).

Focus

We focused entirely on fifth grade this time.  As readers may recall a couple of weeks back, I spent a couple of days at EMES and the focus of the fifth grade was division (Division, Order of Operations and Ugly Fraction Problem... post).

This time, our goal was to move students from the intuitive to concrete/pictorial to abstract/symbolic understanding of division using the standard algorithm efficiently.  We had three days (Monday, Wednesday and Friday) to spend.  For each of the three days that I was there, our schedule was the same:

7:45 - 9:00 -     I was available for planning/debriefing.  
9:00 - 10:00 -   I worked in one fifth grade class.
10:00 - 10:30 - The teacher and I debriefed.
10:30 - 12:45 - I was available for meetings.
12:45 - 1:45 -   I worked in the other fifth grade class.
1:45 - 2:15 -     The teacher and I debriefed.

The focus of our collaborative time was looking at the evidence of student understanding collected using formative assessments to decide how to mold our instruction to improve understanding.

Goals

Our eventual goal is to get all fifth grade students to the Applications/Communications level of proficiency for division of multi-digit whole number dividends by multi-digit whole number divisors.  This would mean that students:  

• Can understand division as: repeated subtraction, equal shares, and the area of a rectangle model.
• Can divide multi-digit whole numbers using the standard algorithm.
• Can explain the standard algorithm using place value and the distributive property of multiplication terminology and through the area of a rectangle model.
• Can contextualize and decontextualize division problems to apply the concept to both routine and non-routine real world problems.

Monday

Robin's students using the manipulatives to approach the entry card.

On Monday morning, we developed an all purpose (entry/exit card) formative assessment for division.  Included on the exit card was:  language containers for division (dividend, divisor and quotient), expressing a division problem in standard algorithm notation, and the area of a rectangle model.  In retrospect, I would have also collected information about whether or not the student could create a context.  I created a new version of this card that I will include under the "Resources" section below.

To introduce the card, we told them that it doesn't "count" as a grade, that they should not feel stressed out by it.  We told them that this is a formative assessment and that means that we are just using it to help us figure out what they understand and what they don't so that we can be more efficient at teaching.  The first day some of the students were worried about the things they could not do, but we told them not to worry that we know that eventually they will be able to do it all with ease.

In one class, we could see from the entry card that students did not have the language containers, many were unsure how to convert to the standard algorithm recording notation. One student was using the standard algorithm using the "Dead Mice Smell Bad" method, but could not explain why.  No one was able to use the area model to conceptualize the division.  Students had Cuisenaire rods and base 10 blocks available to them but opted not to use them.

We decided to look at the problem "48 divided by 10" to start out.  Emphasizing the language and that what we are trying to do is:

"Build the largest rectangle we can that has an area of 48 and a vertical length of 10."

At the end of class, we had students revisit the same problem on the entry card.  Below is an example of one student's growth.

This student made progress with the language and syntax and has an intuitive sense of division as the reverse of multiplication.  He is unsure how to represent it as the area of a rectangle and record it using the algorithm.

There were enough students in this class who needed reinforcement of the language piece  that she decided to focus on language building (and tying the language to the area of a rectangle model) the next day. 

In the other class, since students had been able to spend more time on division, we decided to devote some class time to working on an understanding of fractions using Cuisenaire rods so that students could interpret remainders as fractions.

When we looked at the entry cards, we found that most students had the language and syntax down.  Students dove right into the Cuisenaire Rods and Base 10 blocks to model the problem.  Many of them had some idea that the area corresponded with the dividend and the vertical length corresponded with the divisor.  They were unsure how place value came into play.

We worked through the problem "127 divided by 10" using the approach and questioning articulate in this instructional plan: Area of A Rectangle Division Instructional Plan.

After that we switched to some Tool Building that involved interpreting the Cuisenaire lengths as variable, the definition of "one whole" keeps changing, forcing us to confront what 3 parts out of 5 represents.  Here is the instructional plan for that: Cuisenaire Linear Fraction Models.  We really focused on having students communicate their reasoning and restating the reasoning that others used (a la Math Practice 3 - Construct viable arguments and critique the reasoning of others).

In retrospect, we wished that we had waited on that until Wednesday and spent the time on a couple more long division examples because on her exit cards, we saw that many students thought the divisor would always be 10 (as in our example).

Tuesday

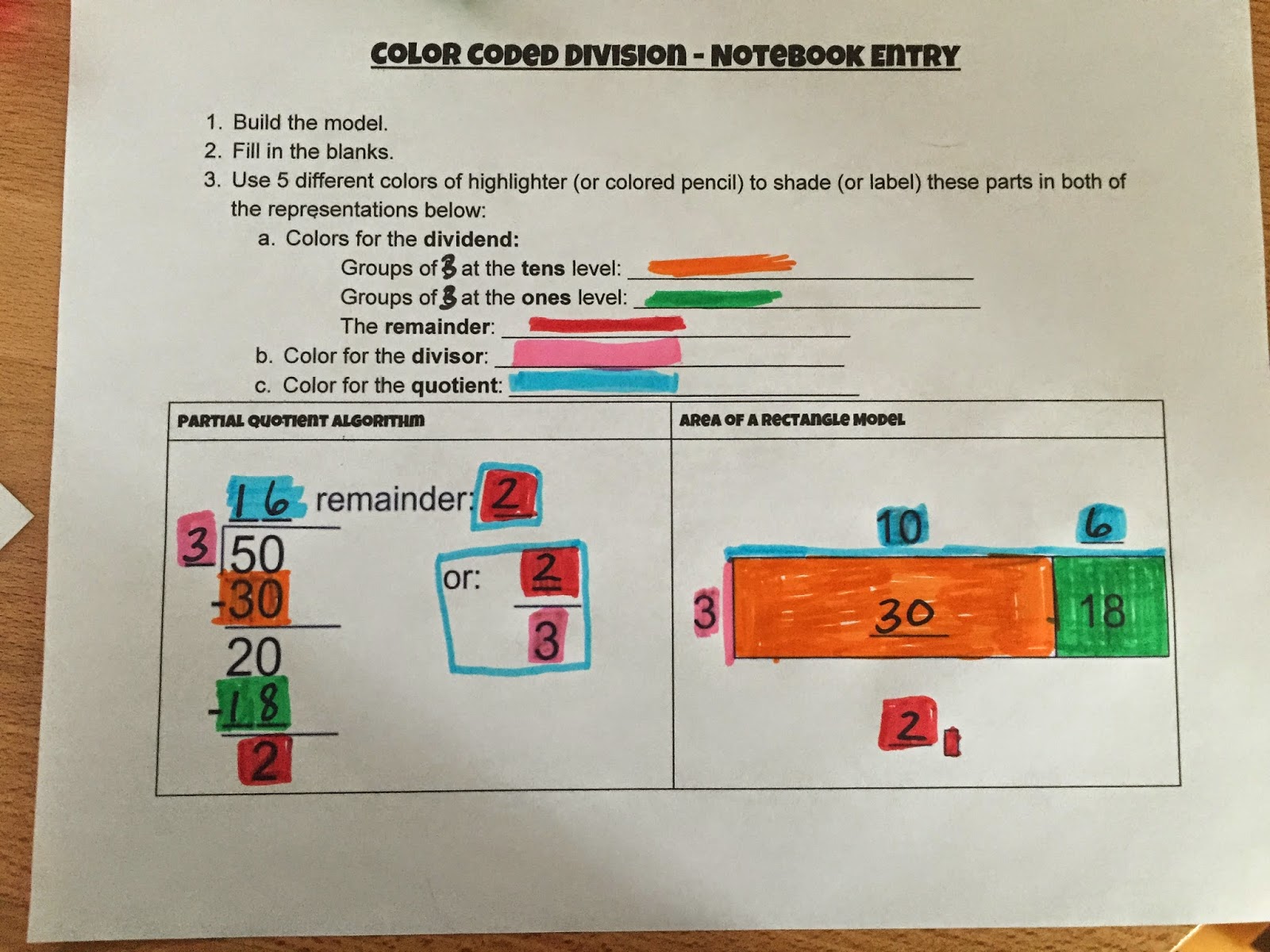
Some students spent time working on developing language.  Other students had made the link between the concrete and the algorithm.  So we decided to have these students work at their own pace on the 5th Grade Division Area Model Marathon to practice and work on getting to the communication level.

Students who had the language, but were shaky on the connection between the model and algorithm worked on this Color Coded Division notebook entry:

 These students built the model.  Then filled in the blanks and color coded the diagram with markers (I recommend matching the colors to the concrete materials) and algorithm to show which parts corresponded with which.  Then these students began working on the Area Model Marathon, too.

Wednesday

In one class, we created another formative assessment, this time with a two digit dividend.  So we decided to start off with that and then do a little bit of the Cuisenaire fractional lengths tool building.  From there we would work on looking at 48 as a dividend using different divisors.

After class, we looked at the formative assessment and decided that:

• The work on language seemed to have helped; and
• There were a few students with whom we needed to follow up, questioning their reasoning.  Such as:

Student A's work

Following up with Student A:  We found out that the student could make the area model, but didn't understand it and "skip counted" by twos to find the quotient.

Student B's work.

Following up with Student B: We found that the student was skip counting by twos to find the quotient and was also having trouble with place value and was not interpreting the "4" in the dividend as "40." 

Neither of these students receive interventions, so we decided that we would need to do some Tool Building and formative assessment around place value on Friday.

In the other class, we started off with more of the work interpreting Cuisenaire lengths as fractions.  Students got really good at explaining their reasoning and proof.  I wish I had thought to record some of them!

We worked through some examples from the concrete to the abstract/symbolic.  I provided a mild, medium and spicy option.  A few students have the area model and algorithm down, so I explained to them that the next piece is being able to work through a problem using place value and the distributive property to explain how the algorithm represents the area model.  

At the end of class, three students were working together on their explanations.  Once they figure it out, they will pair up with students who need more explicit examples and test out their communication knowledge. 

Friday

In one class thought we might use the color coded division entry, but realized that some  students who need it might not be ready for the three digit dividend.  We decided that I would make a two digit version that could be used with 4th grade students and also as an option for fifth grade students who are not yet comfortable with the three digits.

We started off with a little Tool Building on place value.  I wrote a 5 digit number on the board and we talked about digits, place value, the value the digits represent and expanded forms of a number.  Then I handed each of them a piece of paper and asked them to express a 6 digit number in semi-expanded and expanded form.  We found three students to follow up with (two of these students do not receive any interventions).

Then we continued with some oral Tool Building using the Cuisenaire rods to represent fractions.  We had a few new folks who had been out sick who found this a little mind blowing.  The teacher did a great job stepping in and keeping one frustrated little guy from giving up by questioning him doggedly as he reasoned through one of the more challenging problems in front of the whole group. 

Then we worked through the problem "48 divided by 2."

We introduce the concept and word: divisibility.  The teacher will be using an exploration of divisibility to work in lots of practice on the standard algorithm while progressing to a new concept that makes work with fractions more efficient.

Finally, we had students reflect on: one thing they learned and one thing that is still confusing and collected it as an exit card.

In the other class, we started off with more of the Tool Building work using Cuisenaire rods to model and interpret varying wholes.  I explained to them that it's important for them to learn that "one whole" depends on how it is defined and that they are working on becoming more flexible in how they think about "one whole."  I also told them that they were actually doing some algebra thinking about the Cuisenaire rods this way, and they looked proud of themselves.

Then, we modeled "2257 divided by 7."  I told the students:

"I'm not going to build this one concretely.  I have built enough of these and worked really hard so that I am able to have the area model in my head while I do the algorithm.  Do you want to see what that looks like?"

The students humored me and said, "yes."  So I drew the area model one place value at a time while I worked through the algorithm.  Then the students worked on their Division Area Model Marathon and all of them tried to "build it in their heads" instead of using the concrete objects this time with Robin and me helping them individually.

At the end of class, we had the group reflect on their growth this week.  Here are a few examples of what they said:

Next steps 

Both teachers will continue to move forward in division with their classes working on divisibility simultaneously, mixing in some Tool Building that targets place value and extended multiplication facts (such as 7 x 800, 70 x 80, etc.).  

As students get comfortable with division of a multi-digit division at the abstract/symbolic level, those students should spend time working on applying and communicating that understanding.  This would be an excellent time to use their Chromebooks to document their understanding.  They could record a video of themselves explaining the algorithm (I told one teacher that they could send these to me, if they wanted or we could do a Google Hangout some time).  They should also be encouraged to find ways to apply this reasoning to the world around them.  I will do a separate post on this later since I believe that this is what we will focus on at Calais in a couple of weeks.

Resources

• Division Entry/Exit Card
• Division Questioning Cards  
• Division Instructional Plan
• Cuisenaire Linear Fractions Models Plan
• Color Coded Division - Two Digits
• Color Coded Division - Three Digits
• 5th Grade Division Area Model Marathon
• Divisibility Rules Organizer 
• Divisibility Rules Instructional Plan
• Division Assessment

Next Up

The next full week after vacation, I will be spending the week at Calais working with the fifth and sixth grade.

In the meantime, please let me know if you have any questions or requests, or if you want to schedule a Google Hangout some time soon.  edorsey@u32.org.

And Happy Vacation!